Effect of Varying Muzzle Velocity and Ballistic Coefficient on a Ballistic App
Introduction
Is it a good idea to adjust the input values for both muzzle velocity (MV) and ballistic coefficient (BC) or will this simply lead to predictions more and more at variance with reality ?
Could you trade off a variation in MV for a compensating variation in BC to match the observed trajectory at one range and how would that affect predictions beyond ?
Is it more important to have an accurate value for MV or BC ?
Uncertainties in Muzzle Velocity
Nobody quite knows how accurate a cheap optical chronograph like the “shooting chrony” really is. There’s no way to test it except by comparison with another instrument. We are typically interested in differences in average MV of 20 – 50 f/s and for reloading applications even down to 5 f/s in extreme spread of single shots. A difference of 25 f/s is 1% of 2500 f/s or 4mm over a typical distance between sensors, which corresponds to the device being 2.2 degrees out of line. Whether a device that costs $200 can measure velocity to within 1% would also need validating.
Uncertainties in Ballistic Coefficient
There will be some uncertainty of measurement in testing actual bullets on the range and the BC also varies with velocity. This calculation depends on measuring velocities at muzzle and down range, so BC has the uncertainty inherent in MV, plus atmospheric variation and undoubtedly others I’m not aware of.
In addition, BC varies with bullet velocity, so the applicable BC can vary depending on the muzzle velocity and range (Figure 1). In principle, a ballistic app could take this into account, if the changes in BC with velocity can be programmed into it, but this is unusual.
Uncertainties in Trajectory
We have an intuitive appreciation that the uncertainty in measurement of mean point of impact (MPI) is comparable with the size of a three shot group and that the long term MPI almost always lies within the spread of a 5 shot group. So, our estimation of the trajectory is limited by the accuracy of our total shooting system, including rifle, ammunition, mirage, shooter and wind. Errors in parallax management could potentially generate bias even greater than the group size, particularly at shorter ranges. Group size, in MOA or milliradians (mRad), tends to increase at longer ranges. This is a significant limitation on verifying actual trajectory at long ranges. This collection of accurate trajectory data is a major benefit of using a more accurate rifle for long range shooting.
Methods
Choice of scenarios:
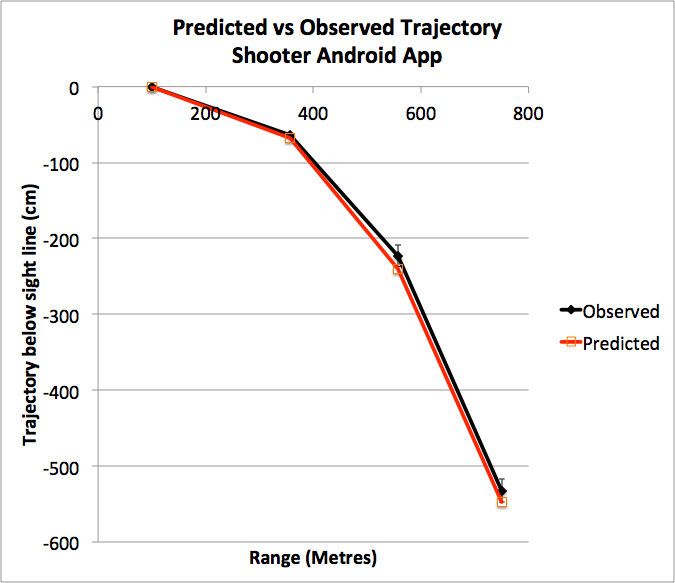
The reference scenario is the trajectory I have verified for my own 7mm08 using 140gr Nosler Ballistic Tip bullets. The parameters of MV = 860 m/s and G7 BC = 0.214, have given reasonably accurate trajectory predictions in practice. BC was estimated from data presented by Brian Litz in “Applied Ballistics for Long-Range Shooting 2 Ed 2011”, for 150gr and 120gr NBT , assuming a similar form factor and calculated sectional density for the 140gr bullet. The MV was estimated by trial and error, finding the velocity which fitted most closely to my observed trajectory in a trial at 100, 300 and 500m. Verification data was collected by shooting at 100m, 400 yd, 600 yd and 800 yd (Fig 1).
I chose variations well outside the usual uncertainties inherent in estimating MV and BC. The MV variant scenarios are 30 m/s (100 f/s) above and below, comparable to chopping off or adding a few inches to the barrel. The BC scenarios are 5% above and 10% below the realistic reference values, corresponding to stepping up to 150gr and down to 120gr Nosler Ballistic Tip bullets respectively
Next, I explored whether changes in muzzle velocity could compensate for ballistic coefficient and still match the trajectory predictions at different ranges. Again, the reference trajectory is from my own 7mm 08 load, verified out to 750m (800 yd). I entered reduced and increased ballistic coefficients into the ballistic app and then adjusted the velocity to give the same elevation at 500m. The zero range was 100m. A cross wind of 5 m/s was entered, to investigate effects on wind drift. The output data were the elevation and windage at 300m and 750m, expressed in mRad. The reduced BC was equivalent to that of an 87 gr 6mm bullet and the increased BC was that of a 168 gr 7.62mm bullet.
Ballistic program
The app used was the Android “Shooter” sold by Kennedy Development Group LLC https://play.google.com/store/apps/d...dy.shooterfull
Figure 1. Variation in Ballistic coefficient with bullet velocity. This shows that experimentally derived BC values may vary by up to 0.008 (2.6%) over the working range of velocities for a commonly used long range 7mm bullet (3000 down to 1500 f/s). Bullet Data - Applied Ballistics, LLC Acc 15 June
2015
Figure 2. Trajectory predicted by Shooter app for 7mm08 using MV 860 m/s and G7 BC 0.214 (red line). The observed trajectory (black line) is for Nosler Ballistic tip 140 gr, AR2208 43.0 gr, Sako 85, barrel length 570mm. The error bars show the observed group sizes at each range. The load was tested and safe in this rifle. Please use starting loads in the ADI manual and work up from there.
Results
Elevation and Windage with Variations in MV and BC
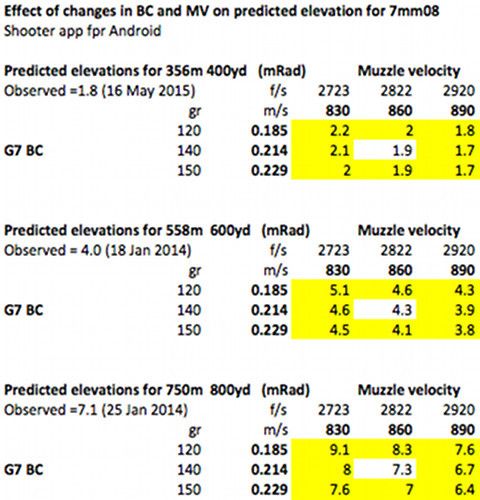
Table 1. Elevation. The verified reference scenario is shown in the central clear cell and predicted required elevation adjustments for variant scenarios are in the yellow cells.
Note: These combinations of Muzzle Velocity and Ballistic Coefficient will not be safely achievable in real 7mm08 rifles and are presented for simulation illustration purposes only.
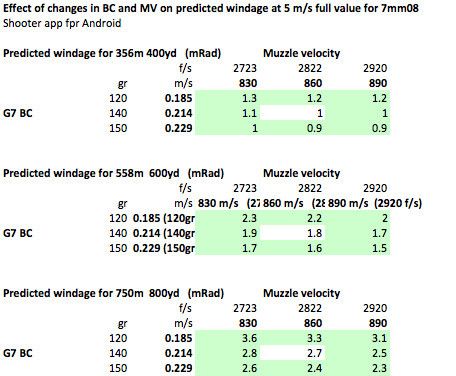
Table 2. Predicted required adjustment for windage.
Illustration of scenarios for 558m (600 yd)
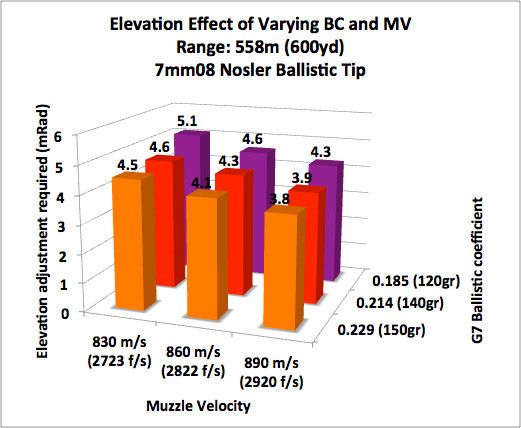
Figure 3. Elevation adjustments from table 1. illustrated for 600 yd.
Typical group sizes for hunting rifles are 0.3 mRad dia (1 MOA). At 558m, trajectory differences corresponding to an MV input error of 30 m/s will just exceed the group diameter. Likewise, a BC difference of 5%, corresponding to 10 gr of bullet weight, is less than typical group diameters.
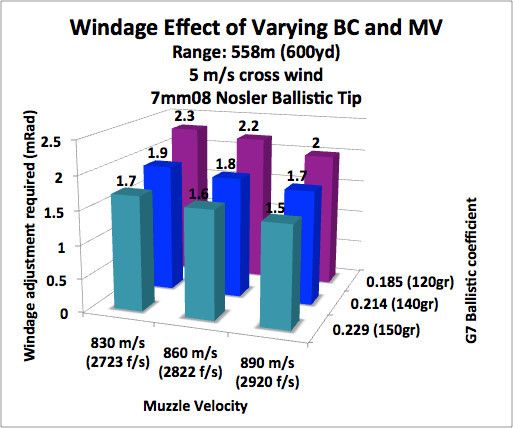
Figure 4. Predicted wind drift at 600 yd.
Velocity differences of plus or minus 30 m/s yield predictions varying by only 0.1 mRad and BC changes corresponding to plus or minus 10 gr bullet weight predict 0.2mRad.
Compensating adjustments to MV and BC
Figure 5 Extreme values of BC were chosen, equivalent to bullets used in different cartridges, then MV was adjusted to compensate and give the same elevation at 500m.
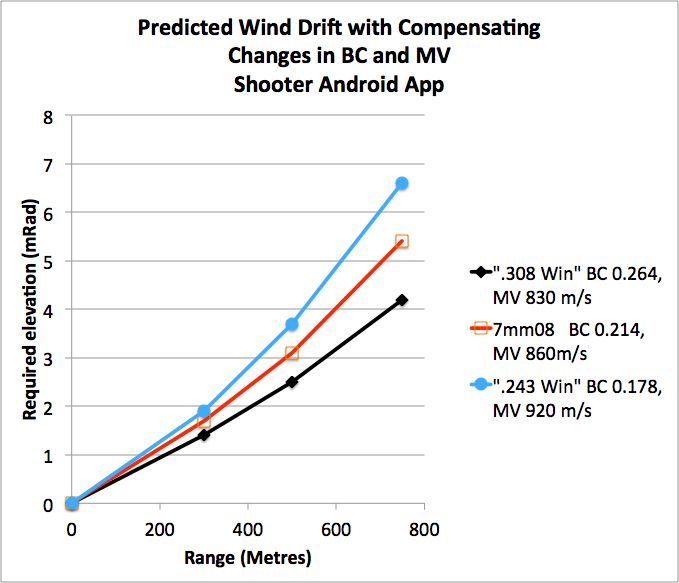
Figure 6. Wind drift predictions for 5 m/s for the BC and MV combinations set up to match elevation at 500m.
Table 3: Exploration of trajectory shape with muzzle velocity and ballistic coefficient adjusted so that trajectory at 500m matches that of the reference 7mm 08 load. MV values were adjusted, to give elevation predictions of 3.5 mRad for 500m. These muzzle velocities corresponded closely to maximum loads listed in the ADI Handloaders’ Manual 2013 for the .243 Win and .308 Win cartridges. Elevation values for 300m were indistinguishable (1.3, 1.4 and 1.4 mRad). It appears possible to develop loads for these three very different cartridges, all based on the .308 Win case, to yield indistinguishable trajectories out to 500m. They differ mainly in their kinetic energy. However, at 750m, elevation values diverged significantly (7.7, 7.3 and 6.9 mRad). The windage was significantly different at all ranges.
Conclusions
On close inspection of tables 1 and 2, the most striking point is that getting 200m closer to the target will roughly halve both the elevation and windage adjustment needed !
At 600 yd (558m), changes in MV of 30m/s (100 f/s), corresponding to around 100mm of barrel length, alter predicted trajectory by around 0.3 Mrad (1 MOA , 15cm or 6 inches), which is comparable to group sizes and uncertainty in measuring actual trajectory for long range hunting rifles.
At 600 yd, changes in BC corresponding to the next higher and lower bullet weights (10 or 20 gr different) alter predicted trajectories by around 0.2 mRad, again comparable to very creditable group diameters for long range hunting rifles.
Alterations to predicted windage requirements are less in angle (mRad) than for elevation, with MV making insignificant contributions to drift. Differences are typically less than 10% of the total windage required and less than 0.2 mRad at 600 yd.
Differences in elevation and windage of around 1 MOA (0.2 to 0.4 mRad) at 600 yd may be accounted for by adjusting either MV and BC. However, the required changes may be substantial, corresponding to a difference in MV of 100 f/s or 10 to 20 gr in bullet weight.
Mismatches of up to 1.5 MOA (0.5 mRad) at 600 yd between observed and predicted trajectories can be reconciled by simultaneous 5% to 10% adjustments to both the MV and BC parameters used in a ballistic App. At 800 yd, such mismatches can be corrected by more modest, realistic tweaks to either MV or BC, especially if both are adjusted to give an additive effect.
Compensating adjustments in MV against BC can be proposed, well outside realistic values, and yield a predicted trajectory indistinguishable from an observed one, out to 500m. However predictions based on unrealistic parameters will fail at longer ranges, such as 750m, as explored here. Windage predictions in these extreme scenarios differ significantly, as close as 300m. So, conventional values of MV and BC should be preferred, where possible. If a frankly extreme combination of MV and BC is to be used, it is worth running the proposed values through a ballistic app and checking elevation on target at the longest anticipated shooting distance. Verifying windage, which is more susceptible to poor modeling, could be very difficult in practice.
If altered values of MV and BC appear warranted, both should be adjusted in the same direction to give an additive effect, while keeping each value within a plausible range, close to those supplied by bullet manufacturers, chronographing or reloading manuals.
Welcome guest, is this your first visit? Create Account now to join.
Welcome to the NZ Hunting and Shooting Forums.
Search Forums
User Tag List
+ Reply to Thread
Results 1 to 15 of 20
-
07-08-2015, 10:53 PM #1Member

- Join Date
- Mar 2012
- Location
- Waikato
- Posts
- 2,188
Adjusting MV and BC to match trajectory
-
-
07-08-2015, 11:44 PM #2
Do you have bryan litz's books as they delve into the mysteries of bullet trajectories ect.
I use litz android program and his validation feature.
It works so i dont fuck with itMy favorite sentences i like to hear are - I suppose so. and Send It!
-
07-08-2015, 11:56 PM #3Member

- Join Date
- Mar 2012
- Location
- Waikato
- Posts
- 2,188
Yes, I've just got Applied Ballistics for Long-Range Shooting 2nd Ed.
He advises against tweaking BC and MV but then his own program does it.
It's real complicated allowing for temp, elevation on different days and so on when you do it yourself. Might be why I can never hit Gillie's 600m gong at Tarata.
I'll bet Litz program does a good job, like an automatic transmission - better than I can.
-
08-08-2015, 12:00 AM #4
Get a sansung s4 phone and it has the sensors to poulate the 3 atmospheres temp humidity and pressure and works it all out.
A way better program than shooter imoMy favorite sentences i like to hear are - I suppose so. and Send It!
-
08-08-2015, 09:21 PM #5
Don't forget to load "weather station pro". The s4 has a thermometer that reads wonky due to the sensors proximity to the battery. If you put that program on, you can offset the error (mine is always 6deg lower). It then figures out DA on a screen widget so you don't need to go digging.
-
08-08-2015, 09:25 PM #6
Also, what scope do you have?. I found out that March fluffed the design of the clicks and used military units instead of what an actual milradian is. Litz used the latter so that's why you need to true the click value to match your real world results. Was relieved to discover I hadn't gone mad after all.
-
08-08-2015, 11:03 PM #7Member

- Join Date
- Mar 2012
- Location
- Waikato
- Posts
- 2,188
Thanks AndyA, I didn't know any phone had those sensors. I must be a techno retro. Google tells me the newer Samsungs dont have the humidity and temp sensors. Maybe they'll bring it back ? I've got a Caldwell wind meter and the temp in that takes ages to stabilise after I get it out of my pocket so it can't be that easy. I'd like to be able to work with density altitude - sounds nice and simple.
DeadIs, this is the scope: FishnHunt - New Zealands Famous Hunting and Fishing Forum Since 1995 - Schmidt and Bender PM II 3-12x50 P P3 . I've tested it to 5 mRad each way but as you can see my grouping wouldn't be good enough to tell if it was actually the NATO 1/6400. However, it says its 1cm so I've just trusted it. You are right; it's best to shoot a group with sights on zero and measure it then adjust and be sure how may click you need at a range.
-
09-08-2015, 10:31 AM #8
Maybe try running it a full 100 clicks and see if it equates to 100cm at 100y. I found I needed to make the click 1.02 to match my hits. I didn't quite understand why litz was recommending .97 be used. In simple terms, I clicked up 100 and only reached 98cm at 100y. By boosting what 1 click value meant (making it 1.02) it seems to track better. You raised the other problem, not being able to hit tight enough to conclusively nail down results. I've stopped going for more than 3 distances in 10 mins. The wind / atmospheric changes over an hour can have you chasing your tail If you try to do too much in one day. The wiser shooters have told me this process can take years to refine. Maybe it's never done, even to the point where your rifle has lost accuracy and you're still chasing that elusive click error....Damn that's pessimistic of me haha.
-
10-08-2015, 11:02 PM #9
Checking the calibration of the scope dials can be done very accurately, so why not eliminate this variable completely?
Something similar to the jig and measuring stick pictured in the thread below is the go.
http://www.nzhuntingandshooting.co.n...tml#post238269
The error in the turret threads are likely large enough to mask whether the milradians are real trigometric, Soviet, NATO, etc. and probably won't be constant throughout the adjustment meaning a correction curve or a lookup table needs to be added to the ballistic program for each scope.
Accurate shooting on the other hand is something I am not qualified to comment on...
-
10-08-2015, 11:21 PM #10
This thread is turning into the old classic saying of,
Out clevering one's selfMy favorite sentences i like to hear are - I suppose so. and Send It!
-
11-08-2015, 09:24 AM #11
Man some guys have plenty of spare time for their hobbies wish I did
-
12-08-2015, 06:50 PM #12
Not sure I follow Andy? Perhaps you or Gibo would be able to advise how MV and BC could be adjusted from observed trajectory data if the scope calibration is not known? The only way I can think of is to leave the scope set at the chosen zero and shoot at a very tall target that records shots 2-3 metres below the point of aim.
Scope turret calibration should not be assumed. It was not so long ago that I measured this on two top-end scopes and both had the elevation running at 71% of the dial setting across the full adjustment.
-
12-08-2015, 08:03 PM #13
I checked my dialling calibration on my nf divari and my vx3 target up 20 moa and they were well with in my shooting ability.
I assume litz,s staggered bcs as been correct and use speed adjustment In the validation mode to get my drops bang on with the real world results.
Seems easier to me to sort the scope calibration than confuse oneself with bc and mv at the same time
I do this on each rifle out to the max distance i shoot and no problems
Works for meMy favorite sentences i like to hear are - I suppose so. and Send It!
-
13-08-2015, 12:58 PM #14
@Puffin, what do I have to do with this?
-
13-08-2015, 01:47 PM #15
Similar Threads
-
How close is your trajectory profile?
By kimjon in forum ShootingReplies: 21Last Post: 09-06-2015, 09:36 AM -
Trajectory data please ?
By Chris in forum Reloading and BallisticsReplies: 11Last Post: 26-09-2014, 06:07 PM -
Adjusting Shooter ballistic program
By Mistral in forum ShootingReplies: 19Last Post: 23-05-2014, 07:49 AM -
Trajectory calculation issue
By distant stalker in forum ShootingReplies: 38Last Post: 03-03-2012, 01:49 AM
Tags for this Thread
Welcome to NZ Hunting and Shooting Forums! We see you're new here, or arn't logged in. Create an account, and Login for full access including our FREE BUY and SELL section Register NOW!!




 14Likes
14Likes LinkBack URL
LinkBack URL About LinkBacks
About LinkBacks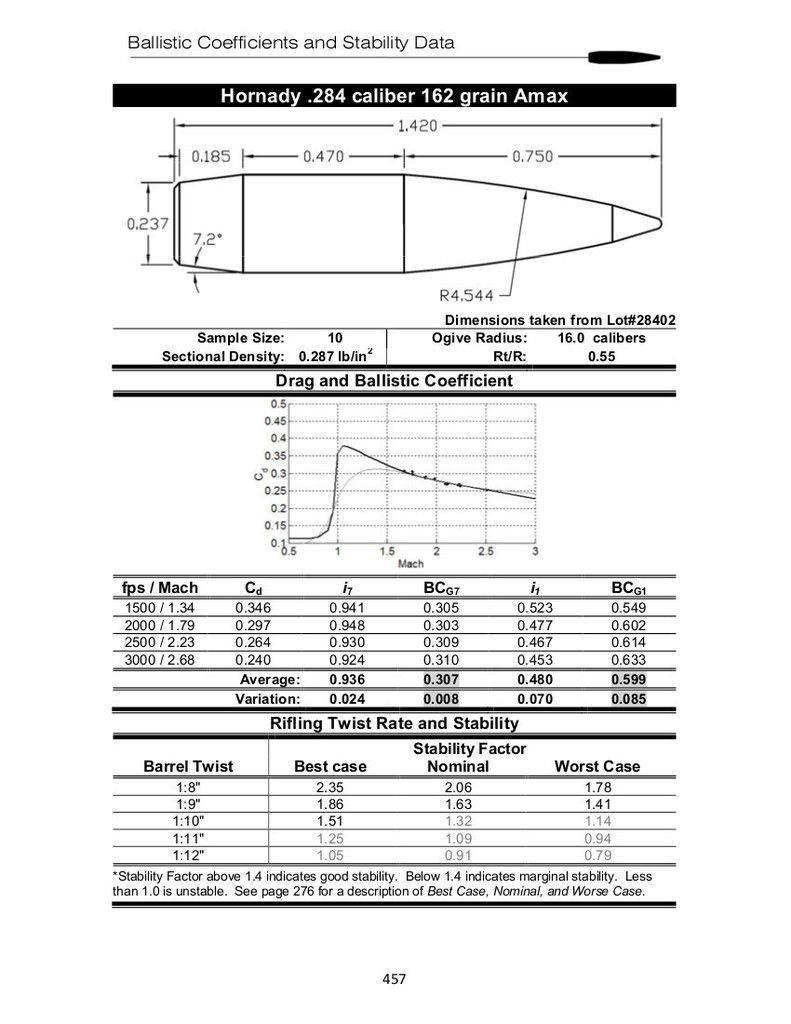





 Reply With Quote
Reply With Quote


Bookmarks